Photonic quantum computers and the new generation of optical quantum sensors require special light and, therefore, special devices.
My research group spent a decade at the University of Hamburg and, before that, a decade at Leibniz University Hannover, pioneering the research and development of a laser system and a measuring device for the second quantum revolution.
Since 2010, a ‘squeeze laser’ from my working group has been in use in the GEO600 gravitational wave detector. Since 2019, my technology has been improving the sensitivities of LIGO and Virgo. The same technology is now commercially available in a compact form. This means that the quantum advantage through quantum correlations can now be transferred to general laser measurement technology and provides the basis for a new light for the photonic quantum computer.
Comparison of the laser light of the first and second quantum revolution
The laser light of the previous first quantum revolution shows no quantum correlations. It is, therefore, neither suitable for photonic quantum computers nor for the new generation of optical quantum sensors. This applies to all previous commercial lasers. The absence of quantum correlations is evident in the measurement: the number of photons, for example, per microsecond of measurement time, can be described by the counting statistics of individual random events.
If quantum correlations are present, the measurement of a first photon is followed by a fixed pattern of further photons. Such a laser light is a prominent figurehead of the second quantum revolution. But how is this laser light produced? And what are the requirements for the measuring apparatus?
Measuring apparatus
The question of the required measuring apparatus is relatively easy to answer. In order for the quantum correlations to be completely reproduced in the measurement signal, each photon must be converted
into a single well-defined electrical signal. This is because missed photons obviously lead to a partially resolved quantum correlation. Similar damage occurs when additional electrical signals are generated without a photon.
A quantum efficiency of close to 100% and a vanishing rate of dark counts are required. One type of measuring apparatus is optimised to measure complex quantum correlations with high optical amplification. These are the so-called balanced homodyne detectors. Other measuring devices are optimised to count individual photon events. These are the so-called single photon detectors.
Production of quantum-correlated, ‘squeezed’ laser light
The different optical quantum technologies require different quantum correlations. In all cases, however, it starts with the generation of the same basic quantum correlation, the ‘squeezed vacuum state’ of a well-defined laser beam. The spatially well-defined laser beam is required so that two such beams can be superimposed with high interference contrast to generate successively more complex quantum correlations. A laser system that generates a light beam in a squeezed vacuum state usually consists of a crystal between two mirrors. The energy source is a very pure pump laser beam.
Fig. 1 shows a photo of these optical components, where the second mirror is deposited directly on the convex polished back of the crystal. The clearly visible pump light here has a wavelength of 532 nm. The special property of the crystal is that the electrically charged components of its atoms cannot follow the oscillations of the green pump light one-to-one. This results in significant oscillation components with half the frequency, which then leads to light with a wavelength of 1064 nm. The quantum correlations result from the fact that, due to the conservation of energy, each photon with a wavelength of 532 nm can only be converted into exactly two photons with a wavelength of 1064 nm.¹
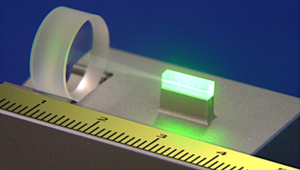
A perfect measuring device will never measure a single photon on this laser beam, as there must always be a second one. Due to induced emission, there can alternatively be four, six, or even a higher number of photons.² Laser light with these quantum correlations is in the so-called ‘squeezed vacuum state’. It is also simply referred to as ‘squeezed light’. The word ‘squeezed’ is a technical term here. It is motivated by the fact that Heisenberg’s uncertainty principle for describing this light can be illustrated not as a circle but as a ‘squeezed’ circle (ellipse). We have suggested calling such a laser system a ‘squeeze laser.’³
The potential of squeezed light and the squeeze laser
- The sensitivity of any laser sensor that already works with very stable conventional laser light can be further improved with squeezed light. This is particularly important if the power of the conventional light cannot be increased any further. In gravitational wave observatories (GWOs)⁴, this is exactly the case because they are operated close to their thermal and opto-mechanical instabilities. In the life sciences and medicine, light power is often limited by the sample. Squeezed light can also help to maintain laser safety.
- Due to the nature of quantum physics, any laser sensor that already uses so much light power that quantum back-action noise is relevant can only be further improved in sensitivity by using squeezed light. This is also the case in GWOs.
- With two squeezed laser beams, quantum cryptography can be raised to a higher security level in optical networks several kilometres in size. With the entanglement generated, not only the communication channel but also the receiver’s measuring devices are mathematically secured.
- Photonic quantum computers, which are universal, fault-tolerant and scalable, require squeezed light as the basis for producing significantly more complex quantum correlations. Without squeezed states, the new and very promising approach of the photonic quantum computer cannot be realised.
Spin-off from the University of Hamburg: Noisy Labs GmbH
Noisy Labs GmbH is the University of Hamburg’s lighthouse spin-off in the field of second-generation quantum technologies. Noisy Labs GmbH is the world’s first company to offer laser systems that generate well-defined laser beams in squeezed vacuum states.
Available wavelengths are 1064 nm and 1550 nm. Fig. 2 shows such a system. The footprint is 60 × 40 cm². Noisy Labs GmbH also offers balanced homodyne detectors with a guaranteed quantum efficiency of more than 95%, whereby the manufacturing chain enables up to over 99% at these wavelengths in principle.⁵

References
- The relationship between the wavelength λ and the frequency f of light results in c=λ∙f, where c is the speed of light. The energy of a photon is proportional to the frequency of the light. E=h∙f, where the proportionality constant h is Planck’s constant
- Photon numbers are specified per measurement time and Fourier-limited spectral width
- R. Schnabel and A. Schönbeck, The “Squeeze Laser”, IEEE Transactions on Quantum Engineering: Quantum Sensing and Metrology 3, 3500209 (2022)
- The LIGO Scientific Collaboration, ‘A gravitational wave observatory operating beyond the quantum shot-noise limit’, Nature Physics 7, 962 (2011)
- H. Vahlbruch, M. Mehmet, K. Danzmann, R. Schnabel, ‘Detection of 15dB squeezed states of light and their application for the absolute calibration of photo-electric quantum efficiency’, Phys. Rev. Lett. 117, 110801 (2016)
Please note, this article will also appear in the 22nd edition of our quarterly publication.









